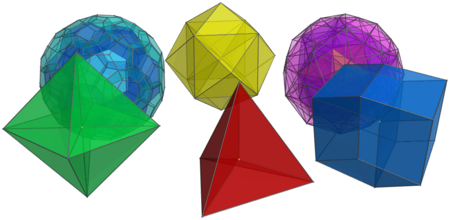
The Regular Polychora

A polychoron is the 4D analogue of a polyhedron in 3D or a polygon in 2D. Just as a 3D polyhedron is bounded by polygonal faces, so a polychoron is bounded by polyhedral cells.

A polychoron is said to be regular if its cells and vertex figures are regular polyhedra. It turns out that there are only 6 possible convex regular polychora in 4D. They are:

Projection images of the regular polychora
[Full-size image]

  1. The pentachoron, shown above in red, also known as the 5-cell, made of 5 tetrahedra, joined 3 to an edge. It is self-dual.

  2. The 4D hypercube, shown above in dark blue, the 4D equivalent of a 3D cube, also known as the 8-cell. It is bounded by 8 cubes, joined 3 to an edge. It is dual to the 16-cell.

  3. The 16-cell, shown above in green, the 4D equivalent of the octahedron. It is bounded by 16 regular tetrahedra joined 4 to an edge. It is dual to the 4D hypercube.

  4. The 24-cell, shown above in yellow, bounded by 24 octahedra, joined 3 to an edge. A unique object analogous to the rhombic dodecahedron, but regular unlike analogues in other dimensions. It is self-dual.

  5. The 120-cell, shown above in cyan, bounded by 120 regular dodecahedra, joined 3 to an edge. It is dual to the 600-cell.

  6. The 600-cell, shown above in magenta, bounded by 600 tetrahedra joined 5 to an edge. It is dual to the 120-cell.

Constructions

The convex regular polychora exhibit inter-relationships with each other that we can use to construct them from each other.

The Tesseract

First of all, the tesseract's coordinates are easily obtained as all changes of sign of:

This gives us a tesseract of edge length 2. Interestingly enough, the radius of each vertex from the center of the tesseract is also 2. This fact will come into play later.

The 5-cell

The 5-cell occurs as the facets of a 5-dimensional cross polytope, whose coordinates are all permutations of coordinates and changes of sign of (1,0,0,0,0). Thus, the coordinates of the 5-cell in 5D are all permutations of:

with no changes of sign. A 5-cell with edge length 2 therefore has coordinates (√2,0,0,0,0) with all permutations thereof. One of the possible ways to project these coordinates back to 4D yields the coordinates:

This is an origin-centered 5-cell with edge length 2.

The 16-cell

The 16-cell cell is the dual of the tesseract, and so can be constructed by finding the centroids of each of the tesseract's 8 cells and taking their convex hull. This yields the coordinates as all permutations and changes of sign of:

The 24-cell

The 16-cell exhibits another connection with the tesseract that may not be obvious at first. Just like the cube, the tesseract's vertices can be colored red or black such that every edges only connects a red vertex to a black one. That is, the tesseract can be alternated. We can then discard either the red vertices or the black vertices, and the resulting shape will have 8 vertices. It turns out that this shape is actually a 16-cell. As in the 3D case, there are two possible cases: taking all vertices (1,1,1,1) with an even number of negative signs, or taking all vertices (1,1,1,1) with an odd number of negative signs. Each yields a 16-cell in a different orientation. Notice that the radius of the 16-cells' vertices is 2. Take the 16-cell constructed as the tesseract's dual, having coordinates (1,0,0,0) with all permutations of coordinates and changes of sign, and scale it to radius 2: (2,0,0,0), with all permutations of coordinates and sign thereof. We now have 3 16-cells, each with 8 vertices of radius 2 from the center. The convex hull of all 24 vertices is the 24-cell. Thus, the 24-cell's coordinates are all permutations of coordinates and changes of sign of:

Since the 24-cell is self-dual, locating the centers of the octahedral cells described by the above coordinates yields the coordinates of the dual 24-cell as all permutations of coordinates and changes of sign of:

The 600-cell

Since the 24-cell can be decomposed into 3 16-cells, we can color its vertices red, green, or blue according to which 16-cell the vertex belongs to. This coloring is such that every triangular face of the 24-cell will have vertices of three different colors, and we can now impose a cyclic ordering of colors, such as red-green-blue, and construct a coherent indexing of its edges. Then we divide the 96 edges of the 24-cell in the Golden Ratio, φ=(1+√5)/2, according to this indexing, and take the convex hull of the points of division. The result is a semiregular polychoron, the snub 24-cell. Starting from the 24-cell with the coordinates (±1,±1,0,0) and all permutations thereof, the coordinates of the corresponding snub 24-cell has as coordinates all even permutations of:

The 24 icosahedral cells of the snub 24-cell can be augmented with icosahedral pyramids, each pyramid consisting of an icosahedral base and 20 tetrahedra. This yields a polychoron bounded by 600 tetrahedra: the 600-cell. The apices of the icosahedral pyramids coincide with the vertices of the 24-cell dual to the one from which we derived the snub 24-cell, which has the coordinates:

with all permutations of coordinates and changes of sign thereof. These are therefore the coordinates of the 600-cell, along with all even permutations of coordinates and all changes of sign of:

The 120-cell

Having obtained the 600-cell, we take its dual to construct the 120-cell. The coordinates obtained by locating the centers of the 600-cell's tetrahedra and scaling by a factor of 2φ−1 are all permutations of coordinates and changes of sign of:

together with all even permutations of coordinates and all changes of sign of:

Credits

Last updated 13 Mar 2019.

Powered by Apache Runs on Debian GNU/Linux Viewable on any browser Valid CSS Valid HTML 5! Proud to be Microsoft-free

This site is best viewed with ANY browser. You do not need a specific version of a specific browser to view this site; all standards-compliant browsers are fully capable of accessing all the content on this site. No unpopular browsers were harmed in the creation of this site.