The 3,3-Duoprism

---

The 3,3-duoprism is a 4D uniform polychoron in the infinite duoprism family. It is the Cartesian product of two triangles, and is bounded by 6 triangular prisms in two rings of 3 each.

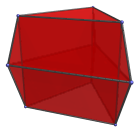
When the two triangles are equal and equilateral, the resulting 3,3-duoprism is uniform and cell-transitive.

Structure

The 6 triangular prisms of the 3,3-duoprism occur in two interlocking rings. The following images show the cells in the first ring:

The following images show the cells in the second ring:

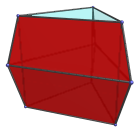
Most of these cells appear squished, because they are seen at an angle. In 4D, they are perfectly uniform triangular prisms.

Animation

The following animation shows the 3,3-duoprism rotating in the plane of its first ring:

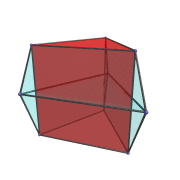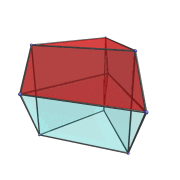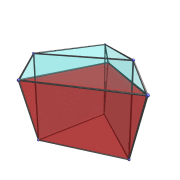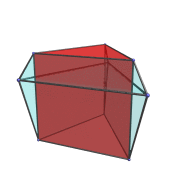
We've colored a single cell red and left the rest in a transparent color so that it's easy to trace the path of rotation.

The next animation shows the 3,3-duoprism performing a double rotation in the planes of its two rings.

This animation shows a peculiarity of 4D, in which an object can simultaneously undergo two mutually orthogonal rotations with independent rates of rotation. Here, the rotation in the plane of the second ring is at 1/3 of the rotation rate in the plane of the first ring.