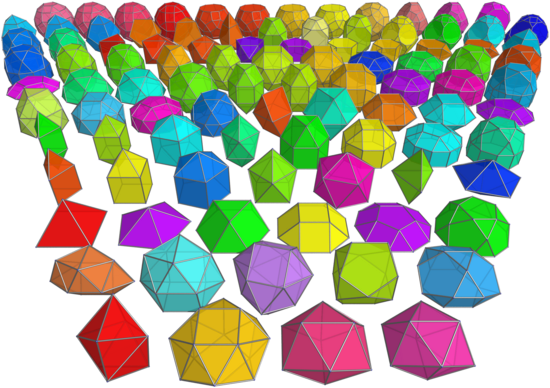
The Johnson Solids

---

The Johnson solids are 3D polyhedra that are convex and have regular polygons for their faces, but are not among the Platonic solids or uniform 3D polyhedra. Norman Johnson enumerated the full list of 92 such polyhedra, which have been named after him.

[Full-size image]

In the above image, 97 solids are shown, because 5 of the Johnson solids are chiral: they are distinct from their mirror images. These are J44–J48. They are paired with their mirror images above, thus making a total of 97 solids.

The Johnson solids occur as cells of the so-called CRF polychora, a generalization of the Johnson solids to 4D.

Pyramids, Cupolae, and Rotundae

The first six Johnson solids are the pyramids, cupolae, and rotundae. A pyramid is formed by adding a point (the apex) over a polygon and erecting triangles connecting the point to the edges of the polygon. A cupola is formed by placing a polygon and its Stott expansion on parallel planes and connecting them with triangles and squares. A rotunda is a hemispherical dome-shaped polyhedron; there is only one in Johnson's list: the pentagonal rotunda, J6.

• The square pyramid (J1);
• The pentagonal pyramid (J2);
• The triangular cupola (J3);
• The square cupola (J4);
• The pentagonal cupola (J5);
• The pentagonal rotunda (J6);

Modifications of J1–J6

The next solids in Johnson's list are formed by pasting together various combinations of the first six plus some prisms and antiprisms. The list is quite long due to combinatorial explosion.

The prefix ortho- means two parts are in matching orientation; whereas gyro- means one part rotated with respect to the other. Thus, an elongation or gyroelongation is the lengthening of a polyhedron by attaching or inserting a prism or antiprism, respectively. A fastigium is a roof-like shape, referring to a triangular prism in J26.

• The elongated triangular pyramid (J7);
• The elongated square pyramid (J8);
• The elongated pentagonal pyramid (J9);
• The gyroelongated square pyramid (J10);
• The gyroelongated pentagonal pyramid (J11);
• The triangular bipyramid (J12);
• The pentagonal bipyramid (J13);
• The elongated triangular bipyramid (J14);
• The elongated square bipyramid (J15);
• The elongated pentagonal bipyramid (J16);
• The gyroelongated square bipyramid (J17);
• The elongated triangular cupola (J18);
• The elongated square cupola (J19);
• The elongated pentagonal cupola (J20);
• The elongated pentagonal rotunda (J21);
• The gyroelongated triangular cupola (J22);
• The gyroelongated square cupola (J23);
• The gyroelongated pentagonal cupola (J24);
• The gyroelongated pentagonal rotunda (J25);
• The gyrobifastigium (J26);
• The triangular orthobicupola (J27);
• The square orthobicupola (J28);
• The square gyrobicupola (J29);
• The pentagonal orthobicupola (J30);
• The pentagonal gyrobicupola (J31);
• The pentagonal orthocupolarotunda (J32);
• The pentagonal gyrocupolarotunda (J33);
• The pentagonal orthobirotunda (J34);
• The elongated triangular orthobicupola (J35);
• The elongated triangular gyrobicupola (J36);
• The elongated square gyrobicupola (J37);
• The elongated pentagonal orthobicupola (J38);
• The elongated pentagonal gyrobicupola (J39);
• The elongated pentagonal orthocupolarotunda (J40);
• The elongated pentagonal gyrocupolarotunda (J41);
• The elongated pentagonal orthobirotunda (J42);
• The elongated pentagonal gyrobirotunda (J43);
• The gyroelongated triangular bicupola (J44);†
• The gyroelongated square bicupola (J45);†
• The gyroelongated pentagonal bicupola (J46);†
• The gyroelongated pentagonal cupolarotunda (J47);†
• The gyroelongated pentagonal birotunda (J48);†

†The last five of these Johnson solids, J44–J48, are chiral: they are distinct from their mirror images. These are the only chiral Johnson solids.

Prism Augmentations

Next are augmentations of the polygonal prisms. An augmentation is the attaching of one or more smaller polyhedra (usually pyramids or cupolae) to a larger one. There is some overlap between this category and the previous one, but we list them separately here because their 4D analogues, the duoprism augmentations, form their own category of CRFs.

• The augmented triangular prism (J49);
• The biaugmented triangular prism (J50);
• The triaugmented triangular prism (J51);
• The augmented pentagonal prism (J52);
• The biaugmented pentagonal prism (J53);
• The augmented hexagonal prism (J54);
• The parabiaugmented hexagonal prism (J55);
• The metabiaugmented hexagonal prism (J56);
• The triaugmented hexagonal prism (J57);

Modifications of Uniform Polyhedra

Following this, we come to the augmentations and diminishings of the regular and uniform polyhedra. A diminishing is an ad hoc cutting off of one or more smaller parts from a polyhedron regardless of symmetry (as opposed to truncation, where the cutting is generally done with respect to the polyhedron's full symmetry.)

• The augmented dodecahedron (J58);
• The parabiaugmented dodecahedron (J59);
• The metabiaugmented dodecahedron (J60);
• The triaugmented dodecahedron (J61);
• The metabidiminished icosahedron (J62);
• The tridiminished icosahedron (J63);
• The augmented tridiminished icosahedron (J64);
• The augmented truncated tetrahedron (J65);
• The augmented truncated cube (J66);
• The biaugmented truncated cube (J67);
• The augmented truncated dodecahedron (J68);
• The parabiaugmented truncated dodecahedron (J69);
• The metabiaugmented truncated dodecahedron (J70);
• The triaugmented truncated dodecahedron (J71);
• The gyrate rhombicosidodecahedron (J72);
• The parabigyrate rhombicosidodecahedron (J73);
• The metabigyrate rhombicosidodecahedron (J74);
• The trigyrate rhombicosidodecahedron (J75);
• The diminished rhombicosidodecahedron (J76);
• The paragyrate diminished rhombicosidodecahedron (J77);
• The metagyrate diminished rhombicosidodecahedron (J78);
• The bigyrate diminished rhombicosidodecahedron (J79);
• The parabidiminished rhombicosidodecahedron (J80);
• The metabidiminished rhombicosidodecahedron (J81);
• The gyrate bidiminished rhombicosidodecahedron (J82);
• The tridiminished rhombicosidodecahedron (J83);

Crown Jewels

The last few Johnson solids are special cases that cannot be obtained by simple cut-and-paste operations on the uniform polyhedra. They are nicknamed crown jewels because they are rare, difficult to find from first principles, and have unique properties.

• The snub disphenoid (J84);
• The snub square antiprism (J85);
• The sphenocorona (J86);
• The augmented sphenocorona (J87);
• The sphenomegacorona (J88);
• The hebesphenomegacorona (J89);
• The disphenocingulum (J90);
• The bilunabirotunda (J91);
• The triangular hebesphenorotunda (J92).

The last two of these, J91 and J92, are indirectly related to the icosahedron via a special, modified form of Stott expansion. Applying this operation to various 4D uniform polychora produces a sizable number of 4D CRF crown jewels, many of which contain J91 and J92 as cells.

The names of these last few solids as explained by Johnson himself:

If we define a lune as a complex of two triangles attached to opposite sides of a square, the prefix spheno- refers to a wedgelike complex formed by two adjacent lunes. The prefix dispheno- denotes two such complexes, while hebespheno- indicates a blunter complex of two lunes separated by a third lune. The suffix -corona refers to a crownlike complex of eight triangles, and -megacorona, to a larger such complex of 12 triangles. The suffix -cingulum indicates a belt of 12 triangles.