The Cylindrical Cone
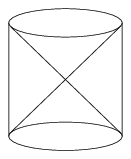
The cylindrical cone, or cylindrone for short, is constructed by tapering a 3D cylinder along the W-axis. The following diagram shows a parallel projection of the resulting object:
This projection shows that the cylindrone consists of a cylinder joined to two cones, which are themselves joined at their apices. The cones look distorted because they are seen at an angle. Besides the cylindrical cell and the two conical cells, there is also a triangular torus formed by the tapering of the round surface of the cylinder along the W-axis. This triangular torus is not obvious in this particular projection; but it is clearer in the next diagram. It may be thought of as the ‘nappe’ of the cylindrone.
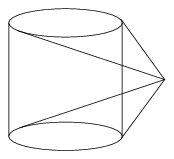
This diagram shows a perspective projection of the cylindrone. Although the two inner cones appear to lie along a straight line, they are actually bent 90 degrees relative to each other in the ZW plane. The region between the two cones and the outer cylinder is the triangular torus we mentioned.
The next diagram shows the cylindrone slightly rotated in the ZW plane.

Its cylindrical cell is now at an angle to the line-of-sight, and hence appears as a frustum. The inner cone is identical to the outer cone, but appears narrower because its base is farther away along the W-axis. The space between the inner and outer cones is the triangular torus, seen at an angle.




