The Spherical Cone
The spherical cone, or spherone for short, is constructed by tapering a unit 3D sphere along the W-axis, analogous to how the 3D cone may be constructed by tapering a unit 2D circle along the Z-axis. The following diagram shows a parallel projection of the spherone:
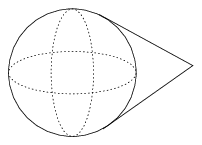
This diagram shows that the spherone consists of a sphere (its “base”) connected to a 3D nappe with the apex at the top. It is the closest 4D analog of the 3D cone.
The next diagram shows the spherone in perspective projection.
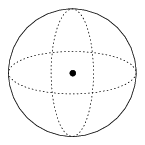
This projection at first glance may not seem to be particularly helpful; however, it may be helpful to realize that the center of this spherical projection is the apex of the spherone. If one had a 3D retina with which to see the 4th dimension, one would see this center “stick out”, and the concentric spherical shells around it taper off linearly in the positive W-axis. This view of the spherone is analogous to looking at a 3D cone apex-on. The 2D projection one sees is a circle with the apex in its center.

This projection shows the spherone slightly rotated in the ZW plane. Its spherical “bottom” appears as an ellipsoid because it is viewed at from an angle.

This projection shows the spherone rotated 90 degrees in the ZW plane. Its spherical base has flattened into a circle, and its projection is now a 3D cone.




