The Triangular Antitegmatic Icosachoron

---

The triangular antitegmatic icosachoron is a Catalan polychoron bounded by 20 cells, 60 faces, 70 edges, and 30 vertices. It is the dual of the runcinated 5-cell.

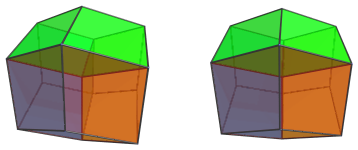
The cells are triangular trapezohedra, which are topologically equivalent to cubes, but with rhombus faces instead of squares. All cells are transitive with each other.

One such cell is shown below:

The red edges are surrounded by 3 cells, whereas the blue edges are surrounded by 4 cells. Notably, all 70 edges of the polytope are all equal length, which is quite unusual for the Catalan polychora. There are 40 edges surrounded by 3 cells, and 30 surrounded by 4 cells.

Of the 30 vertices, there are two kinds: the first kind is the 10 vertices where 4 cells meet; the second kind is the other 20 vertices where 6 cells meet.

The vertex-first projection of the triangular antitegmatic icosachoron resembles the vertex-first projection of the tesseract. However, they are different shapes in 4D, and should not be confused with each other.

Structure

We will explore the structure of the triangular antitegmatic icosachoron using its parallel projections into 3D, centered on a vertex of the first kind, where 4 edges meet.

The Near Side

The following image shows the vertex nearest to the 4D viewpoint:

For the sake of clarity, we render the rest of the polychoron in a light, transparent color.

Surrounding this vertex are 4 triangular trapezoidal cells, shown next:

These are the cells that lie on the near side of the polytope.

The Equator

Next, we come to the equator of the polychoron. There are 12 cells that lie on the equator, shown below:

These cells have been foreshortened into rhombuses, because they lie at a 90° angle to the 4D viewpoint. However, in 4D they are full-bodied triangular trapezohedra. For the sake of clarity, we omit the cells on the near side of the polychoron that we have already seen.

The Far Side

Now we come to the cells on the far side of the polytope. Their layout mirrors the layout of cells on the near side, except in complementary orientation.

These are the cells that lie on the far side of the polytope.

Summary

In summary, there are 4 cells on the near side of the polytope, 12 on the equator, and 4 more on the far side, for a total of 20 cells.

Coordinates

The Cartesian coordinates of the triangular antitegmatic icosachoron are:

• ±(1/√10, 1/√6, 1/√3, ±1)
• ±(1/√10, 1/√6, −2/√3, 0)
• ±(1/√10, −3/√6, 0, 0)
• ±(±2/√10, 2/√6, −1/√3, ±1)
• ±(±2/√10, 2/√6, 2/√3, 0)
• ±(3/√10, −1/√6, −1/√3, ±1)
• ±(3/√10, −1/√6, 2/√3, 0)
• ±(3/√10, 3/√6, 0, 0)
• ±(4/√10, 0, 0, 0)

These coordinates correspond with a dual runcinated 5-cell of edge length 1.