4D Euclidean space
News Archive
Jun 2019
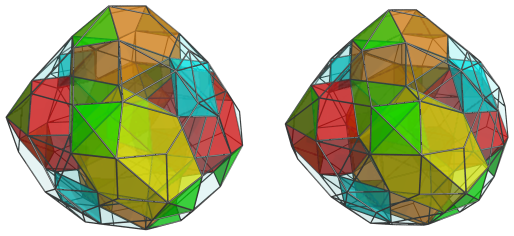
This month we present a very interesting CRF polychoron that sports 24 bilunabirotunda (J91) cells and has demitesseractic symmetry:
This is the pentagonorhombic trisnub trisoctachoron, or pretasto for short, named by Jonathan Bowers. Its surface is bounded by 24 bilunabirotundae (J91), 8 cuboctahedra, 40 octahedra, 32 tridiminished icosahedra (J63), and 40 tetrahedra. It is also known by the designation D4.11, a temporary unique identifier assigned by the Discovery Index of the CRF discovery project.
Find out more at the D4.11 page, which describes its structure in detail, and, as usual, provides the full algebraic coordinates.
18 Jun 2019:
Added vertex, edge, and polygon counts for all polytopes on this website. Partial information was already there before, but now the missing parts are included. If you find any errors in this information, please let us know!
17 Jun 2019:
Fixed a missing ± sign in the Cartesian coordinates of the hebesphenomegacorona (J89). If you notice any other errors on this website, do let us know!
3 Jun 2019:
The Polytope of the Month for June is up!
May 2019
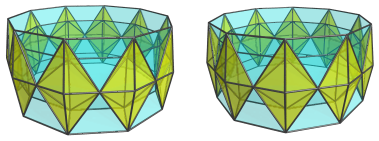
This month we bring you the smaller cousin of last's month Polytope of the Month. In fact, it's the Stott-contraction of the latter, a CRF polychoron called the deca-augmented 5,10 duoprism.
The primary point of interest in this polychoron is its surface that contains 10 pentagonal bipyramids (J13), formed by the pairs of pentagonal pyramid cells of each pair of adjacent augments lying on the same hyperplane and merging into bipyramids. Its other cells are 5 decagonal prisms and 50 square pyramids.
Find out more about this interesting polytope by visiting the deca-augmented 5,10-duoprism page, where we also provide the full Cartesian coordinates, as is customary.
23 May 2019:
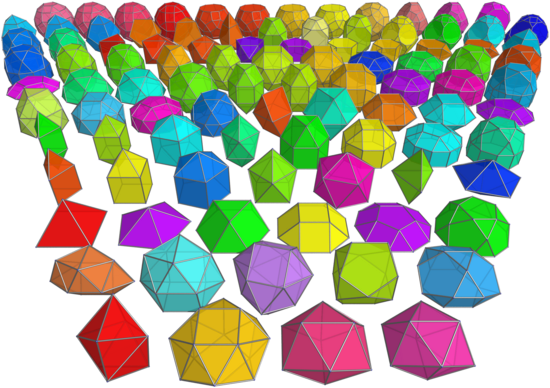
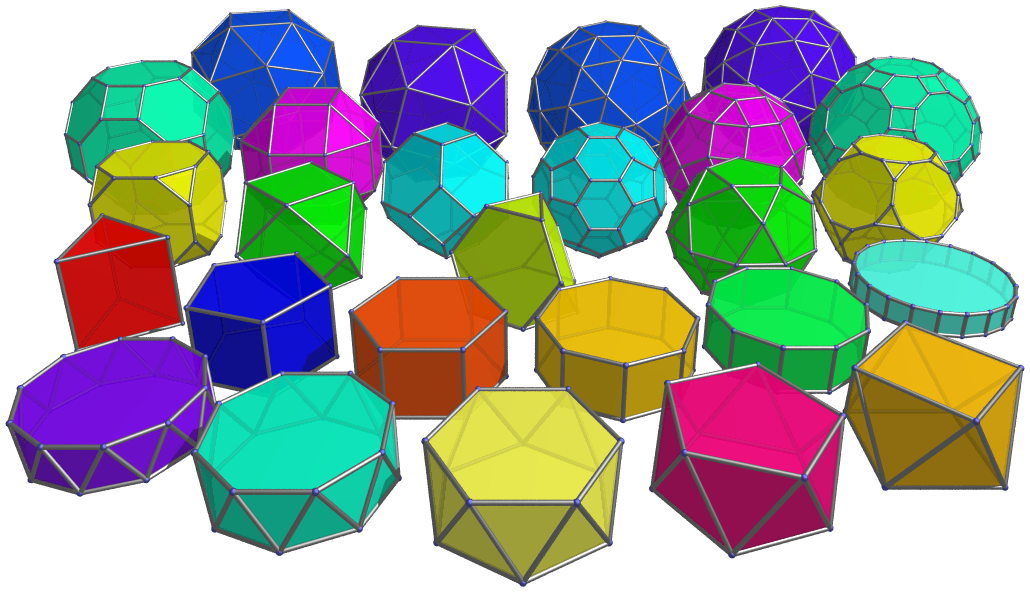
Added a family portrait of the Johnson solids (including the 5 enantiomorphs of the 5 chiral members):
High-resolution version available on the Johnson solids page.
21 May 2019:
Added the bigyrate diminished rhombicosidodecahedron (J79), the 79th Johnson solid.
This completes the list of 92 Johnson solids on this website! Visit the Johnson solids page for the full list. Full algebraic coordinates are provided for every Johnson solid, allowing the numerical computation of the coordinates to arbitrary precision. Enjoy!
17 May 2019:
Added the metagyrate diminished rhombicosidodecahedron (J78), the 78th Johnson solid.
7 May 2019:
Added the paragyrate diminished rhombicosidodecahedron (J77), the 77th Johnson solid.
1 May 2019:
The Polytope of the Month for May is up!
Apr 2019
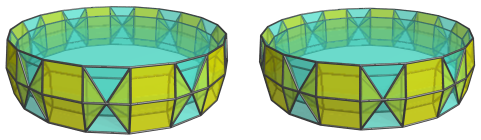
This month, we bring you an interesting CRF polychoron that sports elongated pentagonal bipyramids (J16) and icosagonal prisms as cells:
This is the decaaugmented 5,20-duoprism, an augmentation of the 5,20-duoprism with 10 pentagonal prism pyramids. Due to the angle between the pentagonal pyramid and pentagonal prism cells in the latter, the pentagonal pyramids are coplanar with the adjacent pentagonal prisms on the 5,20-duoprism, causing them to merge into 10 elongated pentagonal bipyramids (J16).
Visit the decaaugmented 5,20-duoprism page for more details about this fascinating polytope, including its full algebraic coordinates.
29 Apr 2019:
Added the trigyrate rhombicosidodecahedron (J75), the 75th Johnson solid.
5 Apr 2019:
Added the metabigyrate rhombicosidodecahedron (J74), the 74th Johnson solid.
1 Apr 2019:
The Polytope of the Month for April is up!
Updated the family portrait of the 3D uniform polyhedra: