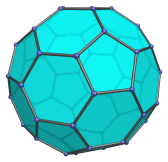
The Truncated Icosahedron

---

The truncated icosahedron is a 3D uniform polyhedron bounded by 32 polygons (20 hexagons and 12 pentagons), 90 edges, and 60 vertices. It is one of the Archimedean polyhedra. It may be constructed by truncating the icosahedron at 1/3 of its edge length, and has 90 edges and 60 vertices.

The truncated icosahedron is widely recognized as the stitching pattern commonly used for soccer balls, and as the shape of the buckminsterfullerene molecule, C₆₀. This latter association has given it the pet name buckyball.

Projections

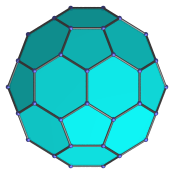
In order to be able to identify the truncated icosahedron in various projections of 4D objects, it is useful to know how it appears from various viewpoints. The following are some of the commonly-encountered views:

Projection	Envelope	Description
	Non-uniform decagon	Parallel projection centered on one of the icosahedral edges (edge shared between two hexagons).
	Icosagon	Parallel projection centered on a pentagon.
	Octadecagon	Parallel projection centered on a hexagon.
	Hexadecagon	Parallel projection parallel to two opposite pentagons.

Coordinates

The Cartesian coordinates of the truncated icosahedron, centered on the origin and having edge length 2, are all even permutations of coordinates and all changes of sign of:

• (0, 1, 3φ)
• (1, 2+φ, 2φ)
• (φ, 2, φ³)

where φ=(1+√5)/2 is the Golden Ratio.

Occurrences

The truncated icosahedron occurs as cells in the bitruncated 120-cell and the cantitruncated 600-cell.