The Cantitruncated 5-cell
The cantitruncated 5-cell is a pretty little uniform polychoron bounded by 20 cells (5 truncated octahedra, 5 truncated tetrahedra, 10 triangular prisms), 80 polygons (20 triangles, 30 squares, 30 hexagons), 120 edges, and 60 vertices.

It can be augmented by the tetrahedral canticupola (K4.76) to make the augmented cantitruncated 5-cell, a CRF polychoron that contains gyrobifastigium (J26) cells.
Construction
The cantitruncated 5-cell can be constructed by expanding the hexagonal ridges of the truncated 5-cell outwards radially. This causes the truncated tetrahedral cells to become truncated octahedra, and the tetrahedral cells to become truncated tetrahedra. The resulting gaps are then filled in by 10 triangular prisms.
Radially expanding the truncated octahedral cells of the cantitruncated 5-cell results in the omnitruncated 5-cell.
Structure
Near Side
We shall explore the structure of the cantitruncated 5-cell by looking at its parallel projection into 3D, centered on a truncated octahedron:

This image shows the nearest truncated octahedron to the 4D viewpoint. For clarity, we render the other cells in a very light color.
The 6 square faces of this nearest cell are joined to 6 triangular prisms, as shown below:

Four of the hexagonal faces are joined to 4 truncated tetrahedra:

The following image shows all these cells together:

These are all the cells on the near side of the cantitruncated 5-cell.
Far Side
The other four hexagonal faces of the nearest cell are joined to 4 other truncated octahedra that lie on the far side of the cantitruncated 5-cell. They in turn surround the farthest cell from the 4D viewpoint, which is a truncated tetrahedron. These cells are shown next:




The green truncated tetrahedron is the farthest cell. The four truncated octahedra appear to be flattened; this is because they are foreshortened by the parallel projection. In 4D, they are perfectly uniform octahedra.
There are 4 other cells on the far side: 4 triangular prisms, which are joined to the triangular faces of the far cell. These are shown below:

These are all the cells on the far side of the cantitruncated 5-cell.
Summary
In summary, on the near side of the cantitruncated 5-cell there are 1 truncated octahedron, 6 triangular prisms, and 4 truncated tetrahedra; and on the far side, there are 4 truncated octahedra, 4 triangular prisms, and 1 truncated tetrahedron. In total, there are 5 truncated octahedra, 10 triangular prisms, and 5 truncated tetrahedra.
Animations
The following animation shows the cantitruncated 5-cell doing a double rotation:
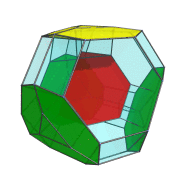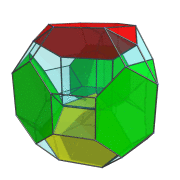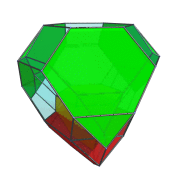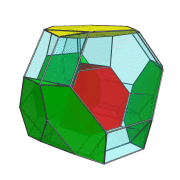
For clarity, we only show the truncated tetrahedral cells. The red and yellow cells appear to be turning inside-out and engulfing each other; but this is merely an artifact of the perspective projection. In 4D they do not touch each other at all.
Similarly, the green cells also appear to be turning inside-out, and at some points intersect with the red and yellow cells. However, this is also just an artifact of the perspective projection. They do not touch each other in reality.
This animation shows a double rotation with two different rates of rotation: the rate of rotation in the plane of the green cells is 1/3 of the rate of rotation in the plane of the red and yellow cells. The double rotation is most obvious with the green cells, which can be seen to be undergoing two types of rotations simultaneously.
Coordinates
The Cartesian coordinates of an origin-centered cantitruncated 5-cell with edge length 2 are:
|
|




