The Swirlprismatodiminished Rectified 600-cell
The Swirlprismatodiminished Rectified 600-cell, also known by its Bowers Acronym spidrox, is a chiral polychoron bounded by 840 cells (120 pentagonal antiprisms, 120 pentagonal prisms, 600 square pyramids), 2640 polygons (1800 triangles, 600 squares, 240 pentagons), 2400 edges, and 600 vertices. It is one of the scaliform but non-uniform 4D polytopes: it is vertex-transitive and has uniform edge lengths. As such, it is also a CRF polychoron.
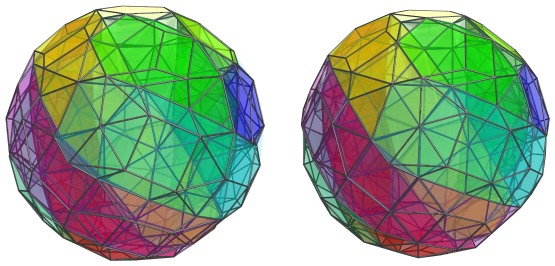
Spidrox may be constructed by deleting 120 vertices lying on 12 great circles from the rectified 600-cell. This diminishing reveals a remarkable substructure of the rectified 600-cell, consisting of 12 rings of alternating pentagonal prisms and antiprisms and 20 helical rings of square pyramids, intertwining with each other in an intricate complex that corresponds with a discrete partitioning of the Hopf fibration of the 4D sphere according to the structure of an icosidodecahedron.
Structure
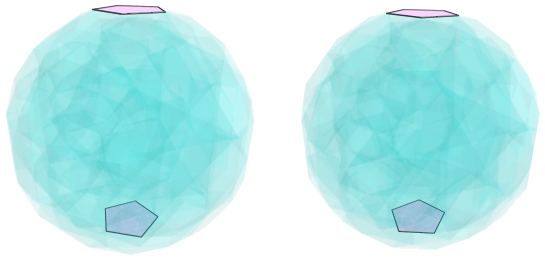
We shall investigate the structure of spidrox using its parallel projections into 3D, centered on one of its pentagonal antiprism cells.
Twelve Rings
The First Ring
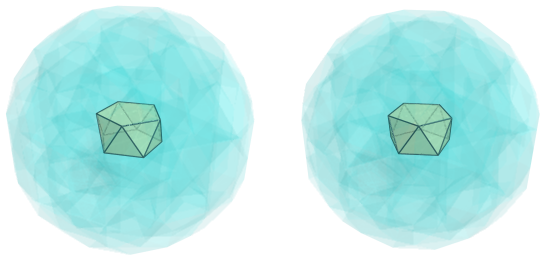
The above image shows the nearest pentagonal antiprism to the 4D viewpoint. For the sake of clarity, we have rendered all the other cells in a light transparent color.
This pentagonal antiprism is joined to two pentagonal prisms, one above, and one below:

On the other side of the pentagonal prisms, are more pentagonal antiprisms:
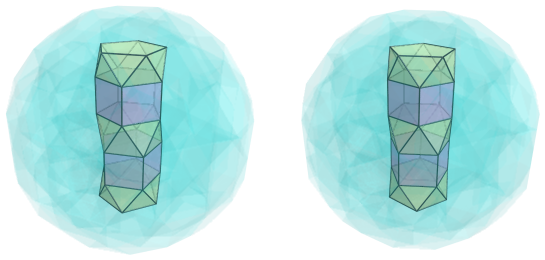
And of course, after them, are another two pentagonal prisms:
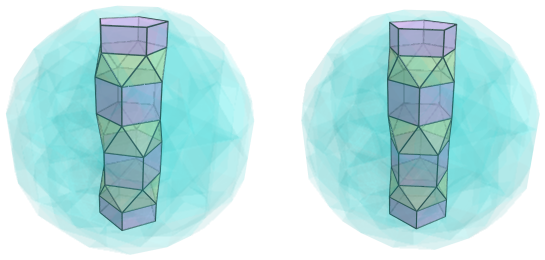
And after those, two more pentagonal antiprisms:

This pillar of alternating cells is actually half of a ring of cells that encircles the polychoron. It appears as a vertical pillar here because the 4D viewpoint is looking parallel to the plane of the ring. The foreshortening of the cells near the top and bottom of the pillar, which makes them look squished, also indicates their curvature into the 4th direction, implying the curvature of the ring.
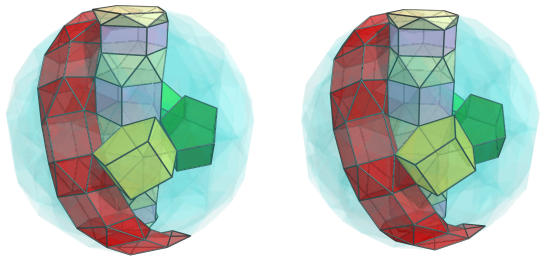
There are 5 pentagonal antiprisms and 4 pentagonal prisms shown here. The top and bottom faces of the pillar are actually the images of two more pentagonal prisms:
The other half of the ring lies on the far side of the polytope, where there are another 5 pentagonal antiprisms and 4 pentagonal prisms:

These cells look identical to the cells on the near side, but they are actually curved in the opposite direction along the 4th dimension, and so join with the latter cells to form a circular ring of 10 pentagonal antiprisms and 10 pentagonal prisms.
The Second Ring
Coming back to the original nearest pentagonal antiprism to the 4D viewpoint:

There are 5 other pentagonal prisms that touch this one at its edges:

Each of these prisms belong to other rings of alternating prisms and antiprisms. For example, the following image shows the ring of cells that the red prism is a part of:
This second ring of alternating prisms and antiprisms continues on the far side of the polytope:
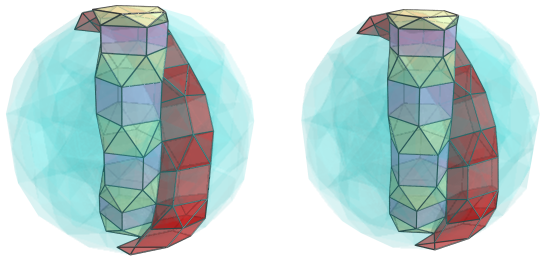
Two pentagonal antiprisms lie on the limb of the projection, linking these two halves together a ring with 10 pentagonal prisms and 10 pentagonal antiprisms. It is, in fact, isomorphic to the first ring, wrapping around it in a spiral fashion.
The Third Ring
As the reader might expect by now, the other pentagonal prisms on the near side are also members of their respective ring of alternating prisms and antiprisms. The following image shows another of these rings:
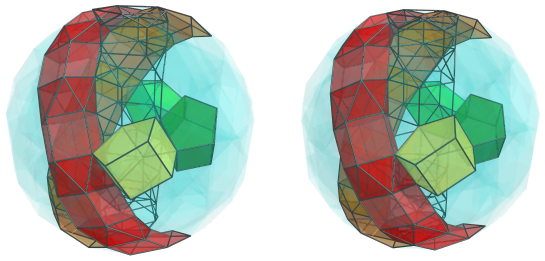
For the sake of clarity, we have rendered the first ring of cells in edge outline only, so that the cells in the third ring would not be obscured.
This is what it looks like on the far side:
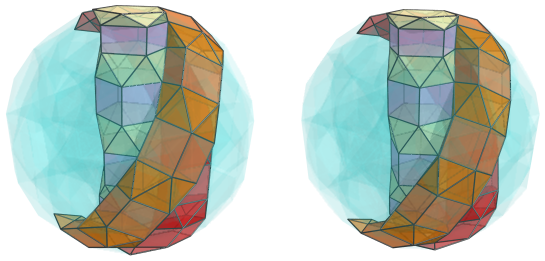
Again, this ring contains 10 pentagonal prisms and 10 pentagonal antiprisms, and is isomorphic to the previous two rings. In fact, its projection image is the same as the projection of the 2nd ring, rotated by 72°. Thus, one might expect that the remaining prisms surrounding the nearest pentagonal antiprism cell in the first ring are also members of more such isomorphic rings. This is indeed the case, as we shall see.
The Fourth Ring
The yellow pentagonal prism that we saw earlier is, as expected, part of a fourth ring of alternating prisms and antiprisms:
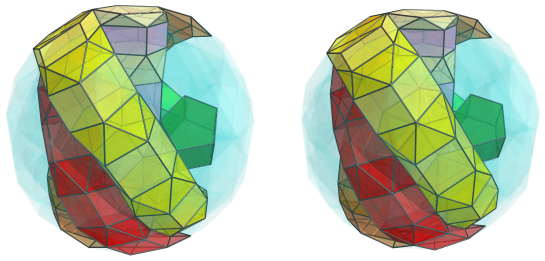
Here are the other cells on the far side of the polytope:
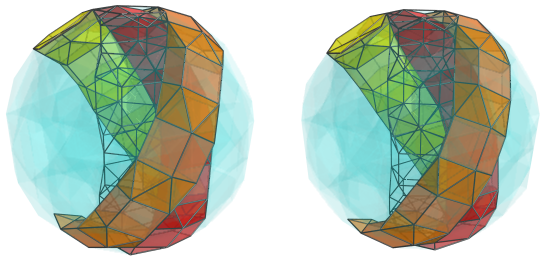
Again, for the sake of clarity, we render the cells in the first ring in edge outline only.
Fifth Ring
The light green prism next to the nearest cell is also part of a fifth ring:
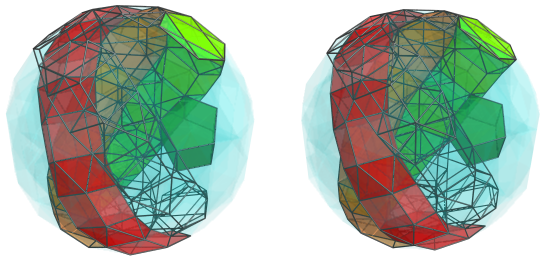
We show rings 1 and 4 only in edge outline, so that the cells in the this ring are less obscured.
Here are the cells on the far side:
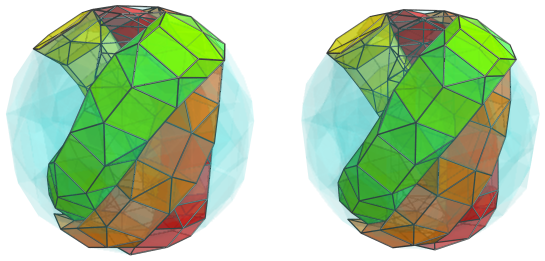
Sixth Ring
The remaining dark green prism next to the nearest cell is, as expected, part of a sixth ring:
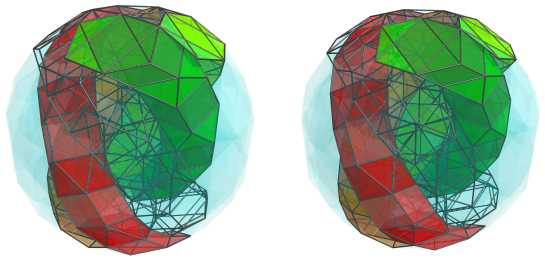
Again, we render the first and 4th rings in edge outline only, in order not to occlude too much of this ring.
Here are its cells on the far side:
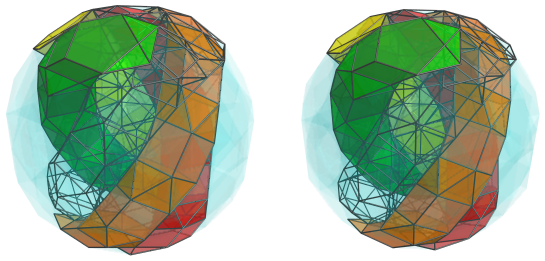
We render the previous, fifth, ring in edge outline only, for the sake of clarity.
These rings all wrap around the first one in a spiralling fashion, as shown below:
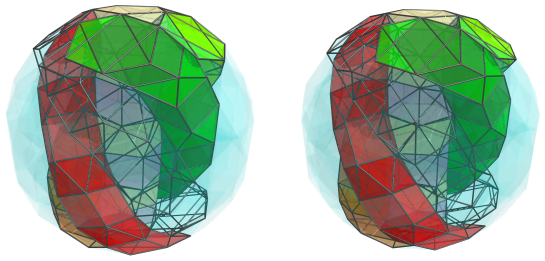
Here are all 6 rings together:
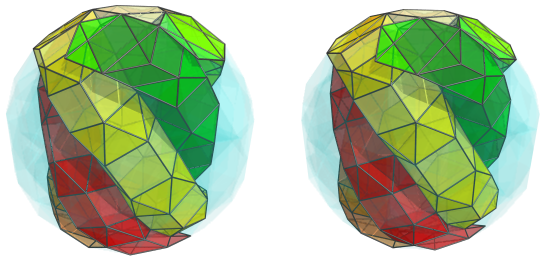
The Seventh Ring
These aren't all the rings yet. As we have mentioned, each of these 6 rings are isomorphic to each other. Just as the first ring have the other five wrapping around it, so each of the other five rings also have 5 rings wrapping around them: two adjacent rings among the 5, the first ring itself, and two new rings that we haven't seen yet. These other rings form an additional layer of rings around the first ring.
The next image shows one of these new rings:
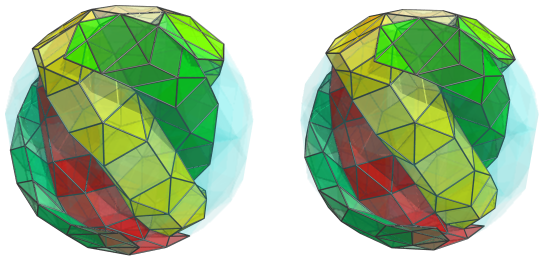
Notice that this ring lies at a shallower angle than the previous rings.
Here's what it looks like on the far side of the polychoron:
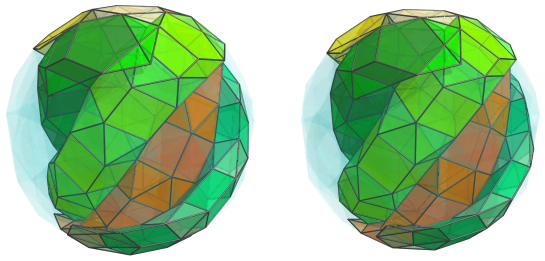
The Eighth Ring
Here is another ring that wraps around the first 6 rings:
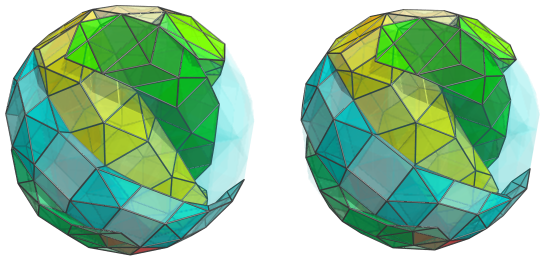
And the far side:
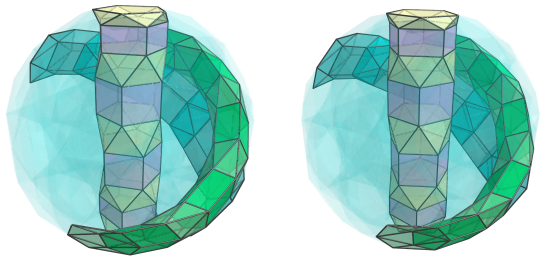
For clarity, we show only the first ring and the 7th ring to serve as a frame of reference, and render all the other cells in a light transparent color.
The Ninth Ring
Here is the next shallow ring:
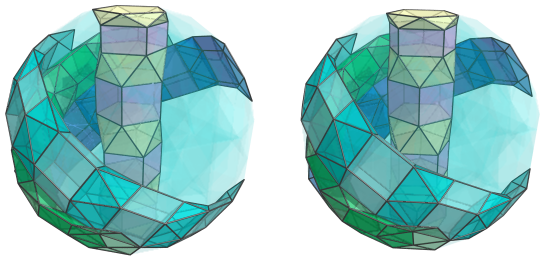
For the sake of clarity, we have omitted rings 2–6, so that they would not obscure this ring.
The far side of this ring:
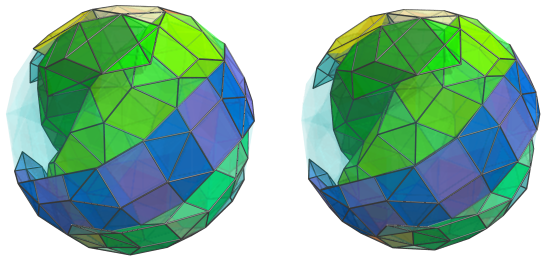
The Tenth Ring
The next ring:
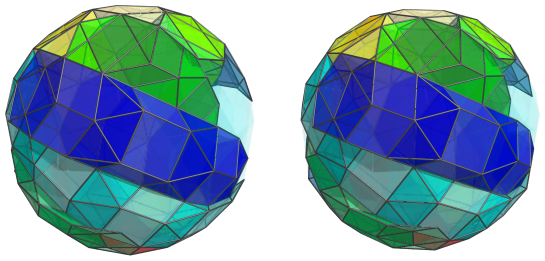
And on the far side:
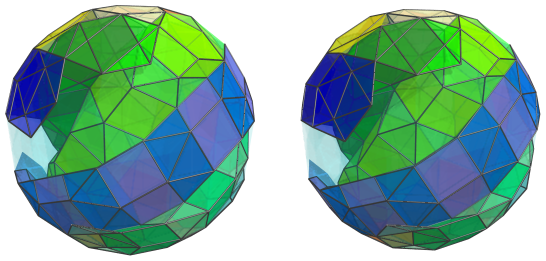
The Eleventh Ring
The last remaining gaps are filled in by the 11th ring:
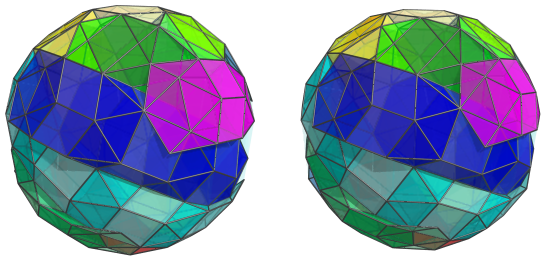
And on the far side:
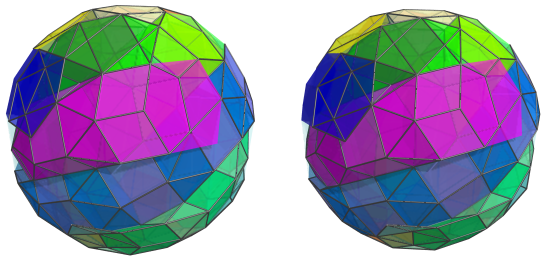
The Twelfth Ring
There is one more ring of alternating prisms in spidrox left. This last ring lies on the limb, or “equator”, of the polytope from this 4D viewpoint:
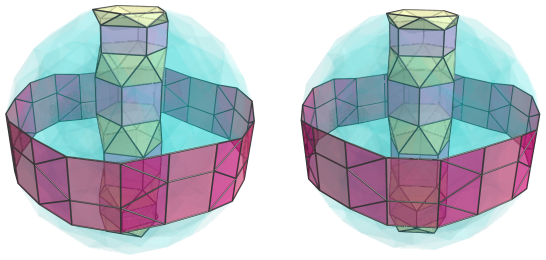
We have omitted all except the first of the previous 11 rings, for the sake of clarity. The prisms and antiprisms appear foreshortened into quadrilaterals because they are seen from a 90° angle. In their native 4D space, they are perfectly uniform pentagonal prisms and pentagonal antiprisms.
As is clearly seen, these two rings lie in mutually orthogonal planes. The following image shows ring 2 and ring 8 included, as representatives of the two layers of rings wrapping around the first ring:
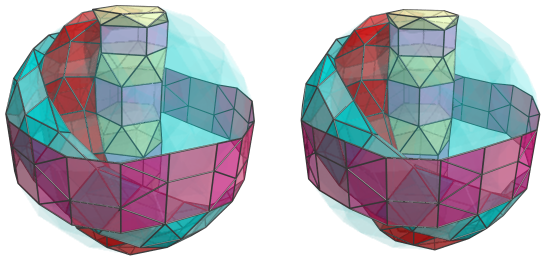
The next image shows the second layer of rings girded by the 12th ring:
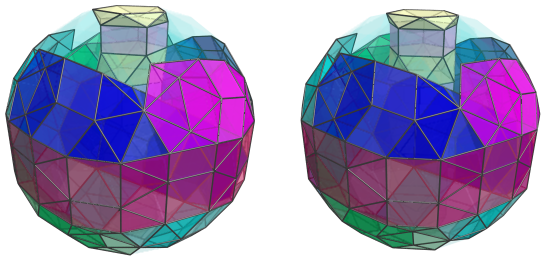
Here are all 12 rings together:
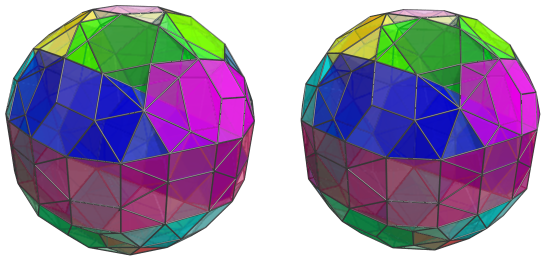
The structure of these 12 rings, interlocking and swirling around each other in a perfectly symmetrical fashion in 4D, corresponds with the discrete partitioning of the Hopf fibration of the 3-sphere derived from the symmetries of the icosidodecahedron. Specifically, each ring maps to a pentagonal face in an icosidodecahedron. Just as each pentagon of the icosidodecahedron touches 5 others at their vertices, so each of these 12 rings touch 5 others at their edges.
Furthermore, where there are triangular faces in the icosidodecahedron, nestled between the pentagons, so in spidrox there are corresponding rings of square pyramids intertwined between the 12 rings of prisms and antiprisms. We look at those next.
Twenty Rings
As we mentioned, all of above 12 rings only touch each other at the edges of the prisms and antiprisms. There are gaps where the square and triangular faces of the prisms and antiprisms lie. These gaps are filled in by 20 rings of square pyramids.
Looking again at the nearest pentagonal antiprism from the first of the foregoing 12 rings:

Its triangular faces are attached to 10 square pyramids:
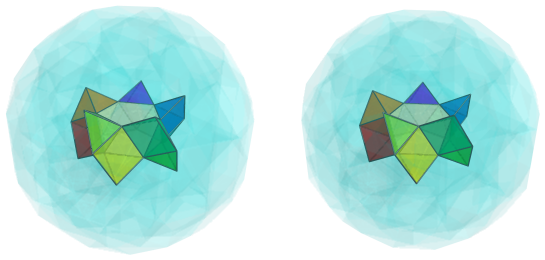
These square pyramids are arranged in a skew fashion in 5 pairs. Each of these 5 pairs of pyramids are actually part of a 30-membered ring.
First Ring
Here is one of the rings of square pyramids around the nearest pentagonal antiprism:
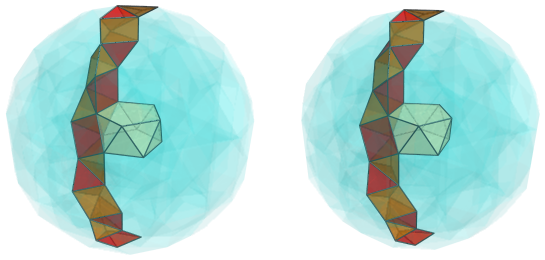
This is the front half of the ring. Here's the back half, on the far side of the polytope:
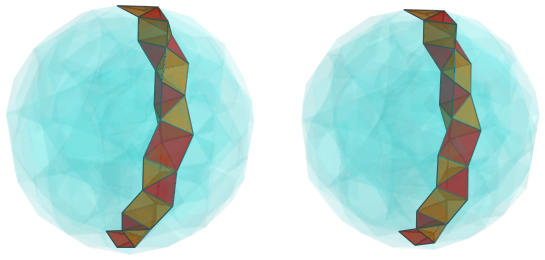
The square pyramids not only form a ring wrapping around the polychoron; they also have a twisting orientation within the ring.
Second Ring
Here's the second ring of square pyramids around the nearest pentagonal antiprism:
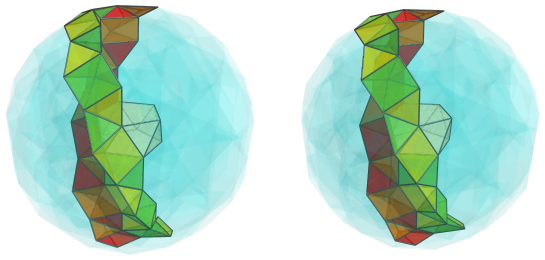
To provide a frame of reference, we leave the first ring visible.
Here is the back half of the second ring:
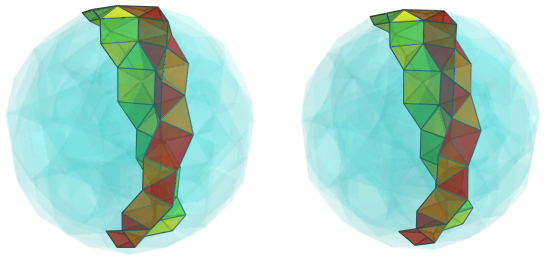
Third Ring
Here's the third ring of square pyramids:
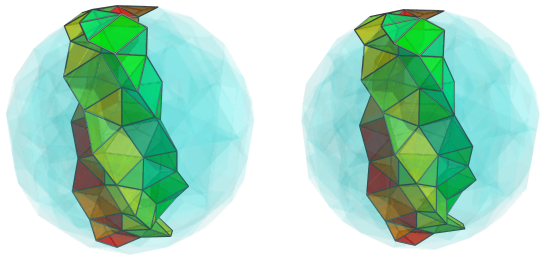
And its back half:
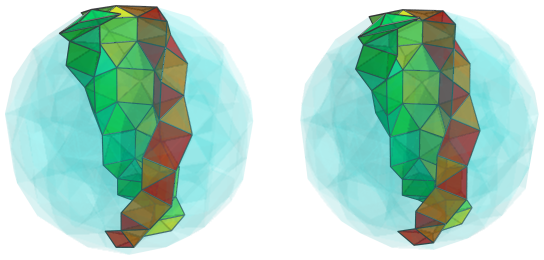
The back view here shows clearly a hollow space between the rings of pyramids where the first ring of prisms and antiprisms would fit.
Fourth Ring
Here's the fourth ring, front and back halves:

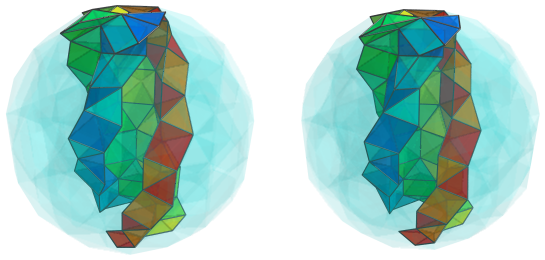
Fifth Ring
Here's the fifth ring, front and back halves:
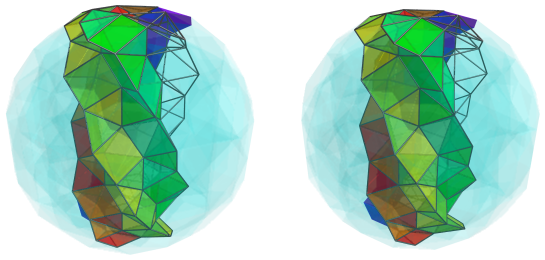
For the sake of clarity, we leave the 4th ring only in outline above.
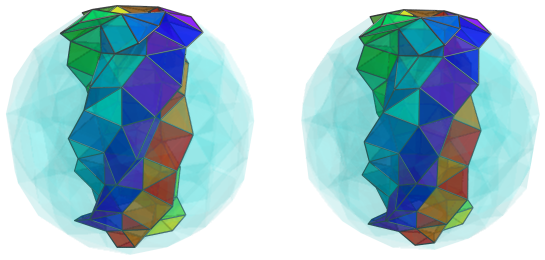
Sixth to Tenth Rings
The foregoing 5 rings of square pyramids are those that swirl around the first ring of prisms and antiprisms. In fact, each ring of prisms and antiprisms has 5 rings of square pyramids winding around it. The second through 6th rings of prisms and antiprisms, for example, each have 5 rings of square pyramids surrounding them, two of which are shared with the first ring, meaning they coincide with two of the foregoing square pyramid rings. Of the remaining 3 rings around each of them, two are shared with the neighbouring rings of prisms among the second through 6th rings, making 5 more rings of pyramids in total.
The image below shows this 2nd layer of 5 rings of square pyramids, the front halves:
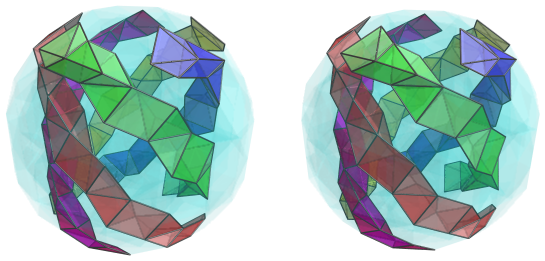
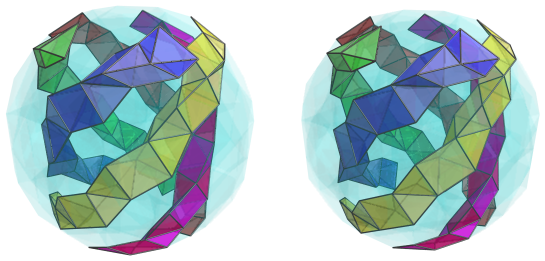
The back halves are shown next:
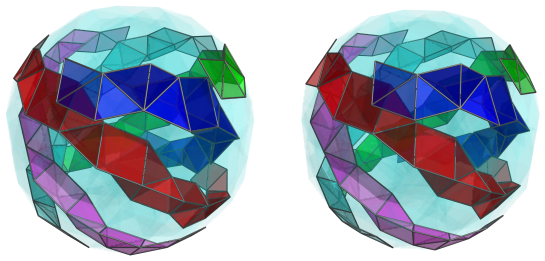
For better reference, here's the front view of all 10 rings together:
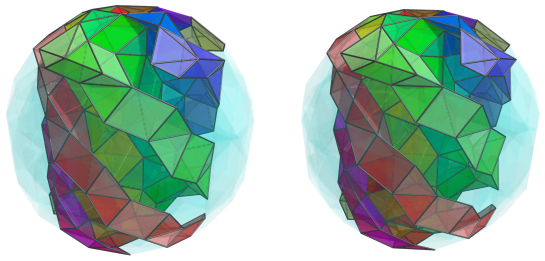
The grooves in this corkscrew-like projection indicate where 5 of the 12 rings of prisms and antiprisms sit.
Eleventh to Fifteenth Rings
Wrapped around the previous 10 rings are another 5 rings, at an even shallower angle. Here are the front halves:
And here are the back halves:
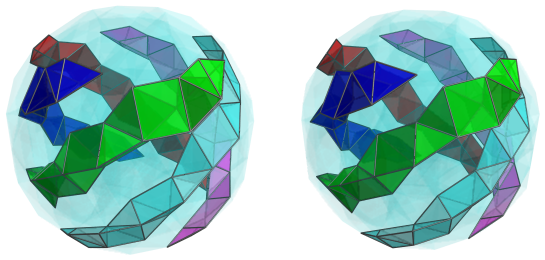
Here are the 5th to 15th rings together, for reference:
Sixteenth to Twentieth Rings
Finally, wrapping around the previous rings are the last 5 rings: the 16th to 20th rings.
Here is the 16th ring, front and back halves:

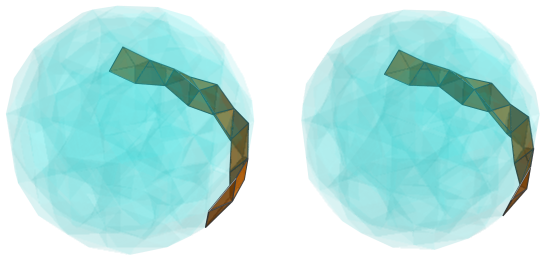
Here is the 17th ring, front and back halves:
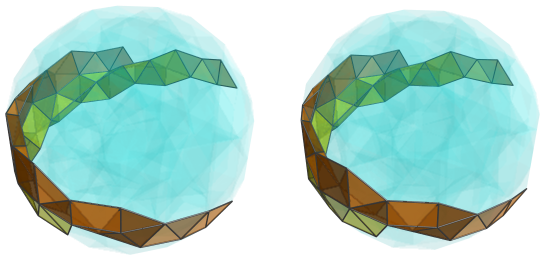
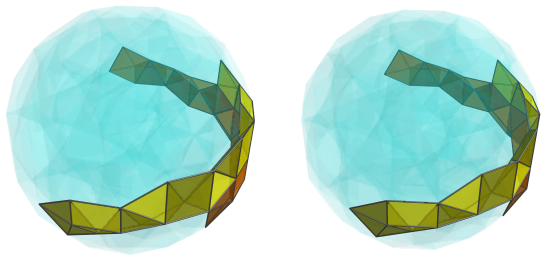
Here is the 18th ring, front and back halves:


Here is the 19th ring, front and back halves:
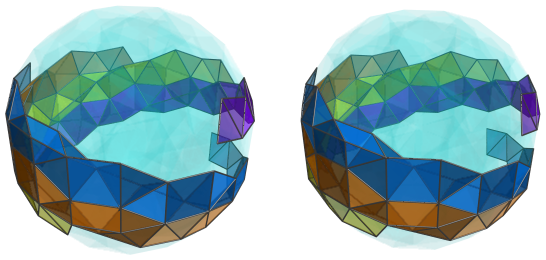
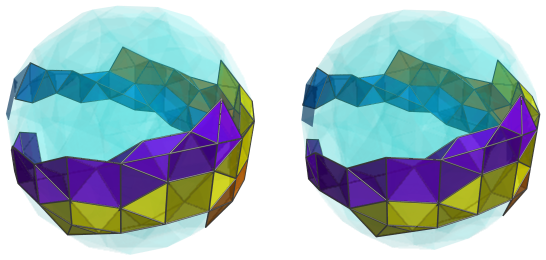
Here is the 20th ring, front and back halves:
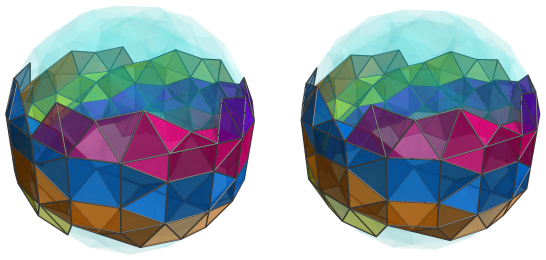
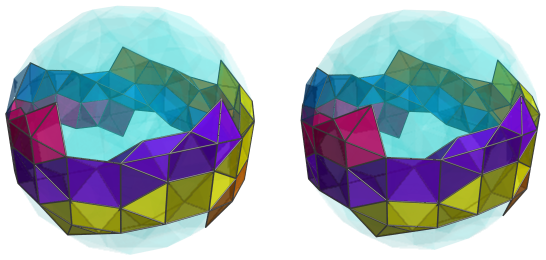
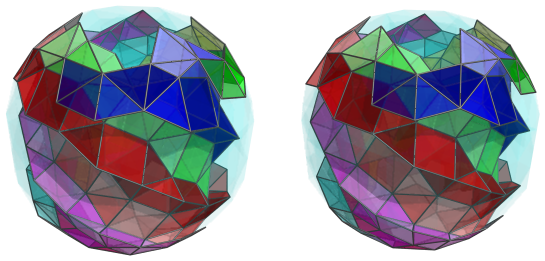
And here are the first 5 and last 5 square pyramid rings together:
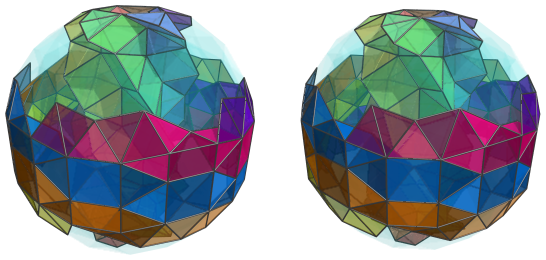
While they appear to be rather different in projection, these two sets of 5 rings are actually identical to each other. These last 5 rings swirl around each other and interlock in exactly the same way as the first 5 rings; the only difference is the angle they make with the 4D viewpoint. In other words, the first 5 rings rotated to the limb of the polychoron would coincide with these last 5 rings, and vice versa.
And indeed, each of the 20 rings of square pyramids are symmetrical with respect to each other. Their relationship with respect to each other is homeomorphic to the 20 triangular faces of the icosidodecahedron. The entire structure of 12 rings of pentagonal prisms and antiprisms and 20 rings of square pyramids corresponds with a discrete partitioning of the Hopf fibration generated by the icosidodecahedron. Just as the triangles and pentagons of the icosidodecahedron share edges, so the prism and antiprism rings share faces with the square pyramid rings. Just as there are 5 triangles surrounding each pentagon in the icosidodecahedron, so there are 5 square pyramid rings surrounding each prism and antiprism ring. Just as the icosidodecahedron can be bisected to form two pentagonal rotunda halves, so spidrox can be partitioned into two identical mutually-orthogonal toroidal bundles of 6 prism and antiprism rings and 10 square pyramid rings.
Summary
The following table summarizes the cell counts of the swirlprismatodiminished rectified 600-cell. PAP refers to the rings of pentagonal prisms and antiprisms; and SP refers to the rings of square pyramids.
The Hopf fibration substructure seen here, in the 12 rings of prisms and antiprisms and the 20 rings of square pyramids, is actually present in all of the 120-cell family uniform polychora, but it is hidden within their higher degree of symmetry. Here, however, the diminishing applied to the rectified 600-cell to produce this polychoron has removed the higher degree of symmetry, leaving this Hopf fibration substructure exposed and obvious.
Coordinates
The coordinates the swirlprismatodiminished rectified 600-cell can be obtained by starting with the coordinates of the rectified 600-cell and deleting from it 12 rings of vertices corresponding with the 12 rings of prisms and antiprisms. This deletes 120 vertices from the rectified 600-cell and yields the 600 vertices of the swirlprismatodiminished rectified 600-cell:
- (0, 0, ±2φ, ±2φ2)
- (0, 0, ±2φ2, ±2φ)
- (0, ±2φ, 0, ±2φ2)
- (0, -2φ, -2φ2, 0)
- (0, 2φ, 2φ2, 0)
- (0, ±2φ2, 0, ±2φ)
- (0, -2φ2, 2φ, 0)
- (0, 2φ2, -2φ, 0)
- (-2φ, 0, 0, 2φ2)
- (2φ, 0, 0, -2φ2)
- (±2φ, 0, ±2φ2, 0)
- (±2φ, ±2φ2, 0, 0)
- (-2φ2, 0, 0, -2φ)
- (2φ2, 0, 0, 2φ)
- (±2φ2, 0, ±2φ, 0)
- (±2φ2, ±2φ, 0, 0)
- (0, -1, -φ, ±(1+3φ))
- (0, 1, φ, ±(1+3φ))
- (0, ±φ, ±(1+3φ), ±1)
- (0, ±(1+3φ), ±1, ±φ)
- (-1, 0, ±(1+3φ), φ)
- (1, 0, ±(1+3φ), -φ)
- (±1, ±φ, 0, ±(1+3φ))
- (±1, ±(1+3φ), ±φ, 0)
- (±φ, 0, ±1, ±(1+3φ))
- (±φ, ±1, ±(1+3φ), 0)
- (-φ, ±(1+3φ), 0, -1)
- (φ, ±(1+3φ), 0, 1)
- (±(1+3φ), 0, ±φ, ±1)
- (±(1+3φ), ±1, 0, ±φ)
- (±(1+3φ), -φ, 1, 0)
- (±(1+3φ), φ, -1, 0)
- (0, -φ2, -φ3, ±(2+φ))
- (0, φ2, φ3, ±(2+φ))
- (0, ±(2+φ), ±φ2, ±φ3)
- (0, ±φ3, ±(2+φ), ±φ2)
- (-φ2, 0, ±(2+φ), φ3)
- (φ2, 0, ±(2+φ), -φ3)
- (±φ2, ±(2+φ), ±φ3, 0)
- (±φ2, ±φ3, 0, ±(2+φ))
- (±(2+φ), 0, ±φ3, ±φ2)
- (±(2+φ), ±φ2, 0, ±φ3)
- (±(2+φ), -φ3, φ2, 0)
- (±(2+φ), φ3, -φ2, 0)
- (±φ3, 0, ±φ2, ±(2+φ))
- (±φ3, ±φ2, ±(2+φ), 0)
- (-φ3, ±(2+φ), 0, -φ2)
- (φ3, ±(2+φ), 0, φ2)
- (-1, -1, ±φ3, -φ3)
- (-1, 1, -φ3, ±φ3)
- (-1, 1, φ3, -φ3)
- (1, ±1, -φ3, φ3)
- (1, -1, φ3, -φ3)
- (1, 1, -φ3, -φ3)
- (1, 1, φ3, φ3)
- (±1, -φ3, -1, ±φ3)
- (±1, φ3, 1, φ3)
- (-1, ±φ3, 1, -φ3)
- (-1, φ3, -1, -φ3)
- (1, -φ3, 1, φ3)
- (1, φ3, -1, φ3)
- (1, φ3, 1, -φ3)
- (±1, ±φ3, ±φ3, ±1)
- (±φ3, ±1, ±1, ±φ3)
- (±φ3, -1, φ3, -1)
- (-φ3, -1, ±φ3, 1)
- (-φ3, 1, -φ3, ±1)
- (-φ3, 1, φ3, 1)
- (φ3, ±1, -φ3, -1)
- (φ3, -1, φ3, 1)
- (φ3, 1, -φ3, 1)
- (φ3, 1, φ3, -1)
- (±φ3, -φ3, -1, ±1)
- (±φ3, φ3, 1, -1)
- (-φ3, ±φ3, 1, 1)
- (-φ3, φ3, -1, 1)
- (φ3, -φ3, 1, -1)
- (φ3, φ3, -1, -1)
- (φ3, φ3, 1, 1)
- (±1, ±φ, ±2φ2, ±φ2)
- (±1, -φ2, φ, -2φ2)
- (-1, -φ2, ±φ, 2φ2)
- (-1, φ2, -φ, ±2φ2)
- (-1, φ2, φ, 2φ2)
- (1, ±φ2, -φ, -2φ2)
- (1, -φ2, φ, 2φ2)
- (1, φ2, -φ, 2φ2)
- (1, φ2, φ, -2φ2)
- (±1, -2φ2, -φ2, ±φ)
- (±1, 2φ2, φ2, -φ)
- (-1, ±2φ2, φ2, φ)
- (-1, 2φ2, -φ2, φ)
- (1, -2φ2, φ2, -φ)
- (1, 2φ2, -φ2, -φ)
- (1, 2φ2, φ2, φ)
- (±φ, ±1, ±φ2, ±2φ2)
- (±φ, -φ2, 2φ2, 1)
- (-φ, -φ2, ±2φ2, -1)
- (-φ, φ2, -2φ2, ±1)
- (-φ, φ2, 2φ2, -1)
- (φ, ±φ2, -2φ2, 1)
- (φ, -φ2, 2φ2, -1)
- (φ, φ2, -2φ2, -1)
- (φ, φ2, 2φ2, 1)
- (±φ, -2φ2, 1, -φ2)
- (-φ, -2φ2, ±1, φ2)
- (-φ, 2φ2, -1, ±φ2)
- (-φ, 2φ2, 1, φ2)
- (φ, ±2φ2, -1, -φ2)
- (φ, -2φ2, 1, φ2)
- (φ, 2φ2, -1, φ2)
- (φ, 2φ2, 1, -φ2)
- (±φ2, -1, -2φ2, ±φ)
- (±φ2, 1, 2φ2, φ)
- (-φ2, ±1, 2φ2, -φ)
- (-φ2, 1, -2φ2, -φ)
- (φ2, -1, 2φ2, φ)
- (φ2, 1, -2φ2, φ)
- (φ2, 1, 2φ2, -φ)
- (±φ2, -φ, 1, 2φ2)
- (-φ2, -φ, ±1, -2φ2)
- (-φ2, φ, -1, ±2φ2)
- (-φ2, φ, 1, -2φ2)
- (φ2, ±φ, -1, 2φ2)
- (φ2, -φ, 1, -2φ2)
- (φ2, φ, -1, -2φ2)
- (φ2, φ, 1, 2φ2)
- (±φ2, ±2φ2, ±φ, ±1)
- (±2φ2, -1, -φ, ±φ2)
- (±2φ2, 1, φ, -φ2)
- (-2φ2, ±1, φ, φ2)
- (-2φ2, 1, -φ, φ2)
- (2φ2, -1, φ, -φ2)
- (2φ2, 1, -φ, -φ2)
- (2φ2, 1, φ, φ2)
- (±2φ2, -φ, -φ2, ±1)
- (±2φ2, φ, φ2, 1)
- (-2φ2, ±φ, φ2, -1)
- (-2φ2, φ, -φ2, -1)
- (2φ2, -φ, φ2, 1)
- (2φ2, φ, -φ2, 1)
- (2φ2, φ, φ2, -1)
- (±2φ2, ±φ2, ±1, ±φ)
- (±φ, ±φ2, ±2φ, ±φ3)
- (±φ, -2φ, φ3, -φ2)
- (-φ, -2φ, ±φ3, φ2)
- (-φ, 2φ, -φ3, ±φ2)
- (-φ, 2φ, φ3, φ2)
- (φ, ±2φ, -φ3, -φ2)
- (φ, -2φ, φ3, φ2)
- (φ, 2φ, -φ3, φ2)
- (φ, 2φ, φ3, -φ2)
- (±φ, -φ3, φ2, 2φ)
- (-φ, -φ3, ±φ2, -2φ)
- (-φ, φ3, -φ2, ±2φ)
- (-φ, φ3, φ2, -2φ)
- (φ, ±φ3, -φ2, 2φ)
- (φ, -φ3, φ2, -2φ)
- (φ, φ3, -φ2, -2φ)
- (φ, φ3, φ2, 2φ)
- (±φ2, ±φ, ±φ3, ±2φ)
- (±φ2, -2φ, -φ, ±φ3)
- (±φ2, 2φ, φ, -φ3)
- (-φ2, ±2φ, φ, φ3)
- (-φ2, 2φ, -φ, φ3)
- (φ2, -2φ, φ, -φ3)
- (φ2, 2φ, -φ, -φ3)
- (φ2, 2φ, φ, φ3)
- (±φ2, -φ3, -2φ, ±φ)
- (±φ2, φ3, 2φ, φ)
- (-φ2, ±φ3, 2φ, -φ)
- (-φ2, φ3, -2φ, -φ)
- (φ2, -φ3, 2φ, φ)
- (φ2, φ3, -2φ, φ)
- (φ2, φ3, 2φ, -φ)
- (±2φ, -φ, -φ2, ±φ3)
- (±2φ, φ, φ2, φ3)
- (-2φ, ±φ, φ2, -φ3)
- (-2φ, φ, -φ2, -φ3)
- (2φ, -φ, φ2, φ3)
- (2φ, φ, -φ2, φ3)
- (2φ, φ, φ2, -φ3)
- (±2φ, -φ2, -φ3, ±φ)
- (±2φ, φ2, φ3, -φ)
- (-2φ, ±φ2, φ3, φ)
- (-2φ, φ2, -φ3, φ)
- (2φ, -φ2, φ3, -φ)
- (2φ, φ2, -φ3, -φ)
- (2φ, φ2, φ3, φ)
- (±2φ, ±φ3, ±φ, ±φ2)
- (±φ3, -φ, 2φ, φ2)
- (-φ3, -φ, ±2φ, -φ2)
- (-φ3, φ, -2φ, ±φ2)
- (-φ3, φ, 2φ, -φ2)
- (φ3, ±φ, -2φ, φ2)
- (φ3, -φ, 2φ, -φ2)
- (φ3, φ, -2φ, -φ2)
- (φ3, φ, 2φ, φ2)
- (±φ3, -φ2, φ, -2φ)
- (-φ3, -φ2, ±φ, 2φ)
- (-φ3, φ2, -φ, ±2φ)
- (-φ3, φ2, φ, 2φ)
- (φ3, ±φ2, -φ, -2φ)
- (φ3, -φ2, φ, 2φ)
- (φ3, φ2, -φ, 2φ)
- (φ3, φ2, φ, -2φ)
- (±φ3, ±2φ, ±φ2, ±φ)
where φ=(1+√5)/2 is the Golden Ratio.




