The Cubical Pyramid
The cubical pyramid is constructed by tapering a 3D cube along the W-axis. It consists of a cubical cell, which is its base, and six square pyramidal cells, its sides, which converge on its apex. It has a total of 7 cells, 18 polygons (12 triangles, 6 squares), 20 edges, and 9 vertices. It is one of the convex, regular-faced polychora.
Parallel Projection
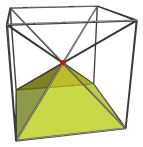
The following image shows an oblique projection of the cubical pyramid:

The cubical base is shown here in green, and the 6 square pyramidal cells in yellow. The square pyramidal cells converge to the apex to the right, shown here in red.
Perspective Projections
The next image shows a perspective projection of the cubic pyramid. This viewpoint happens to look directly at its apex. The projection has a cubical envelope, and forms the boundary of its cubical base.

The apex of the cubical pyramid projects to the center of the cubical volume. The edges extending from this apex to the corners of the cube outline the 6 square pyramids. They are attached at their bases to each face of the cube, with their apices meeting at this apex.
The following images show these square pyramid cells:





The next projection shows the cubical pyramid rotated slightly in the ZW plane. We see that its apex now projects outside its cubical base.
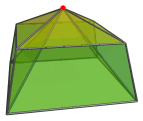
The last projection shows the cubical pyramid rotated 90 degrees in the ZW plane, so that its base lies on the XYW hyperplane.

We see that its cubical base now projects onto a flat square, and it now has a square pyramidal envelope.
The cubical pyramid can also have a hexagonal pyramidal envelope when viewed from yet another angle, as shown below:
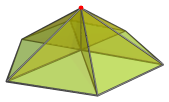
Coordinates
The coordinates of the cubical pyramid with edge length 2 are:
- (±1, ±1, ±1, 0)
- (0, 0, 0, 1)
Geometric Relationships
Eight cubical pyramids can be joined together with their apices sharing a common vertex, to form a tesseract.
Eight cubical pyramids can also be attached at their bases to the cubical cells of a tesseract: the square pyramidal cells pair up to form octahedra, resulting in a 24-cell.
An analogous modification can be done to the runcinated tesseract by attaching 8 cubical pyramids to its axial cubical cells. The square pyramid cells lie on the same hyperplanes as the non-axial cubical cells, and merge into elongated square bipyramids (J15), Johnson solids. The result is the octa-augmented runcinated tesseract, a CRF polychoron.




