The Deltoidal Hexecontahedron

---

The deltoidal hexecontahedron is a 3D Catalan solid bounded by 60 kites, 120 edges (60 short, 60 long), and 62 vertices. It is the dual of the rhombicosidodecahedron.

The faces are transitive kites with 2 short edges and 2 long edges. The edge length ratio is 3√(17−7φ) : 11, or approximately 0.650 : 1, where φ=(1+√5)/2 is the Golden Ratio. The ratio of the short diagonal of a face to its long diagonal is 3(5φ−7) : 2√(11−5φ), or approximately 0.959 : 1.

The 62 vertices are of 3 kinds: 20 vertices where 3 edges meet, corresponding to an inscribed dodecahedron; 12 vertices where 5 edges meet, corresponding to an inscribed icosahedron; and 30 vertices where 4 edges meet, corresponding with an inscribed icosidodecahedron.

Projections

The following are images of the deltoidal hexecontahedron from various viewpoints:

Projection	Description
	Projection centered on an order-5 vertex.
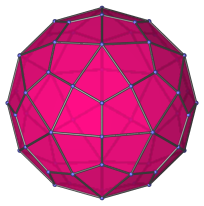	Centered on an order-3 vertex.
	Centered on an order-4 vertex.
	Centered on a face.

Animation

Here's an animation of a deltoidal hexecontahedron rotating around the vertical axis:

Coordinates

The Cartesian coordinates for the deltoidal hexecontahedron are all permutations of coordinate and all changes of sign of:

• (0, 0, 2φA)
• (1, 1, 1)

along with even permutations of coordinate and all changes of sign of:

• (1, 1/φ, φ)
• (0, Bφ, B)
• (A, φA, φ²A)

where φ=(1+√5)/2 is the Golden Ratio, A=(5φ−7)/2, and B=(3φ−2)/3.

These coordinates are obtained by inverting a rhombicosidodecahedron of edge length (8φ−10)/11.