The Octa-augmented Runcitruncated 16-cell

---

The octa-augmented runcitruncated 16-cell is a CRF polychoron with 240 vertices, 768 edges, 768 polygons (320 triangles, 384 squares, and 64 hexagons), and 240 cells (8 octahedra, 160 triangular prisms, 24 elongated square bipyramids (J15), 32 hexagonal prisms, and 16 truncated tetrahedra).

It may be constructed by augmenting the runcitruncated 16-cell with 8 octahedron || rhombicuboctahedron segmentochora (K4.107). The square pyramids of the augments and the cubical cells of the runcitruncated 16-cell lie on the same hyperplane, and merge into elongated square bipyramids. It can also be obtained via a Stott expansion of the octa-augmented runcinated tesseract. Equivalently, it can be considered as a partial Stott contraction of the runcitruncated 24-cell.

Structure

The Near Side

We shall explore the structure of the octa-augmented runcitruncated 16-cell by examining its parallel projections into 3D, centered on one of its octahedral cells:

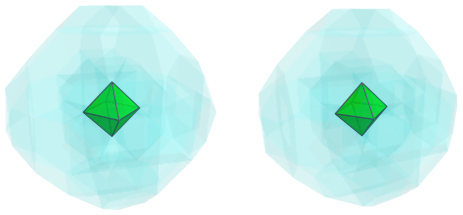
This octahedral cell is surrounded by 8 triangular prisms:

Straddling each adjacent pair of these triangular prisms are 12 more triangular prisms:

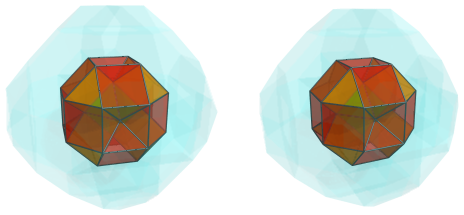
Touching the vertices of the octahedron are 6 square bipyramids:

The square faces of these square bipyramids are joined to 12 hexagonal prisms:

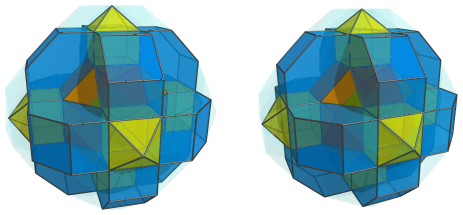
The remaining exposed faces of the triangular prisms are where 8 truncated tetrahedra are fitted:

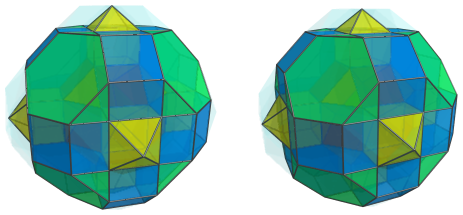
Surrounding the protruding tips of the square bipyramids are florets of triangular prisms in alternating pattern, 8 prisms around each tip, thus totalling 48 more triangular prisms:

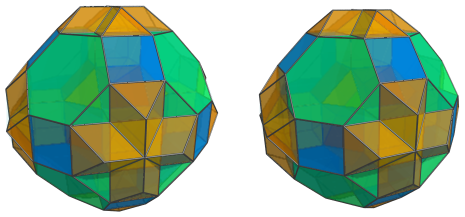
These are all the cells that lie on the near side of the polytope, the side facing the 4D viewpoint. Past this point, we reach the equator of the polychoron.

The Equator

There are 12 square bipyramids that lie on the equator of the octa-augmented runcitruncated 16-cell. They are shown below:

For clarity, we have omitted the cells that lie on the near side of the polychoron.

These square bipyramids lie at a 90° angle to the 4D viewpoint; consequently, they appear foreshortened to hexagons. In reality, they are undistorted J15's.

The tips of these bipyramids touch 6 octahedral cells:

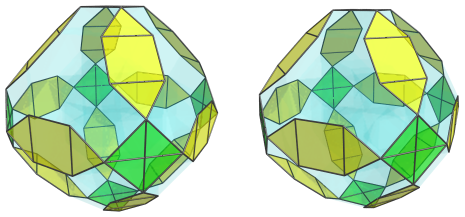
These octahedra have also been foreshortened into squares because they lie at a 90° angle to the 4D viewpoint. They are, of course, perfectly regular octahedra in 4D.

Sharing edges with these octahedra are 24 triangular prisms:

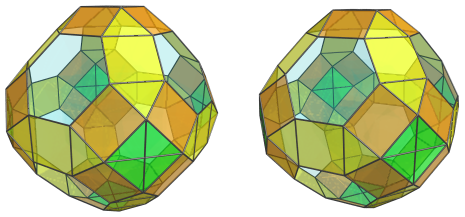
These triangular prisms have been foreshortened into squares because of the 90° angle with the 4D viewpoint.

The remaining hexagonal gaps are closed by 8 hexagonal prisms:

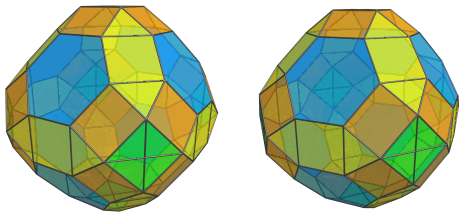
These hexagonal prisms have been foreshortened into hexagons, but in 4D they are, of course, perfectly uniform hexagonal prisms.

These are all the cells that lie on the equator. Past this point, we reach the far side of the polytope, where the arrangement of cells mirrors that of the near side.

Summary

The following table summarizes the cell counts for the various regions of the polychoron:

Region	Layer
Near side	1	1	20	0	0	0
	2	0	48	6	12	8
	Subtotal	1	68	6	12	8
Equator		6	24	12	8	0
Far side	2	0	48	6	12	8
	1	1	20	0	0	0
	Subtotal	1	68	6	12	8
Grand total		8	160	24	32	16
		240 cells

Coordinates

The Cartesian coordinates of the octa-augmented runcitruncated 16-cell, centered on the origin and with edge length 2, are all permutations of coordinates and changes of sign of:

• (0, 0, √2, 2+2√2)
• (1, 1, 1+√2, 1+2√2)