The Pentagonal Antiprism
The pentagonal antiprism is a 3D uniform polyhedron bounded by 12 polygons (2 pentagons and 10 triangles), 20 edges, and 10 vertices.
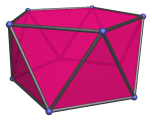
The pentagonal antiprism can be derived from the regular icosahedron by cutting off two opposite vertices. Conversely, we can erect two pentagonal pyramids on the pentagonal antiprism, one on each of its pentagonal faces, and thus obtain the icosahedron.
Projections
In order to be able to identify the pentagonal antiprism in various projections of 4D objects, it is useful to know how it appears from various viewpoints. The following are some of the commonly-encountered views:
| Projection | Envelope | Description |
|---|---|---|
 |
Regular decagon | Parallel projection centered on a pentagonal face. |
 |
Rectangle | Parallel projection parallel to pentagonal faces. |
 |
Parallelogram | Parallel projection centered on an edge shared by two triangular faces. |
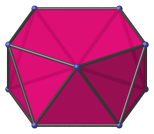 |
Octagon | Vertex-centered parallel projection. |
Coordinates
The Cartesian coordinates of the pentagonal antiprism, centered on the origin and having edge length 2, are:
- ±(√(2(5+√5)/5), 0, √((5+√5)/10))
- ±(√((5−√5)/10), ±φ, √((5+√5)/10))
- ±(√((5+2√5)/5), ±1, −√((5+√5)/10))
where φ=(1+√5)/2 is the Golden Ratio.
These coordinates give a pentagonal antiprism parallel to the XY plane, in a “nice” orientation for placing in 3D scenes.
An alternative set of coordinates can be obtained by exploiting the pentagonal antiprism's connection with the icosahedron. Removing two opposite vertices from the icosahedron and taking the convex hull of the remaining 10 vertices yields the pentagonal antiprism. Taking the origin-centered coordinates for the icosahedron as our starting point, we obtain the following origin-centered coordinates for the pentagonal antiprism:
- ±(0, 1, −φ)
- (±1, ±φ, 0)
- (±φ, 0, ±1)
These coordinates do not yield a nice orientation of the pentagonal
antiprism, but they are algebraically nicer
in that all coordinates can
be expressed in terms of multiples of the Golden Ratio, and do not involve
nested radicals.
Occurrences
The pentagonal antiprism occurs as cells in the grand antiprism.
It also occurs as cells in the following CRF polychora:




