The Rectified Tesseract
The rectified tesseract is a uniform polytope in the tesseract family. It is constructed by truncating the tesseract at the midpoints of its edges, and its surface consists of 24 cells (16 tetrahedra, 8 cuboctahedra), 88 polygons (64 triangles, 24 squares), 96 edges, and 32 vertices.

The dual of the rectified tesseract is the joined 16-cell, a Catalan polychoron.
Structure
The structure of the rectified tesseract is relatively simple. It retains the 8 cells of the tesseract it is derived from, except that these cells been rectified into cuboctahedra. The vertices of the original tesseract have been truncated, leaving tetrahedra in their place.
The following image shows the parallel projection of the rectified tesseract, centered on one of its cuboctahedral cells:

For clarity, we show only the nearest cuboctahedron to the 4D viewpoint. The next image shows the 8 tetrahedra that are joined to this cuboctahedron:

Here's the cuboctahedron together with the tetrahedra:

As can be clearly seen, the projection envelope of the rectified tesseract is a cube. The faces of this cube correspond to the projection images of 6 more cuboctahedral cells, shown in pairs below:



These cuboctahedra appear to be flattened into squares; that's because they lie at a 90° angle to the 4D viewpoint. In 4D, they are perfectly uniform cuboctahedra.
On the far side of these cuboctahedra are another 8 tetrahedra and the antipodal cuboctahedron to the first cuboctahedron we saw. The arrangement of these cells is identical to the arrangement of the previous 9 cells on the near side. The following sequence of images shows how all the cells of the rectified tesseract are laid out in the parallel projection:







This layout shows clearly that there are 8 cuboctahedral cells in total, along with 16 tetrahedral cells.
Animations
The following animation shows the rectified tesseract rotating in the WX plane:
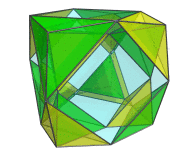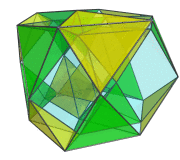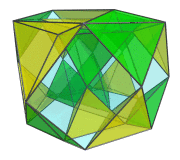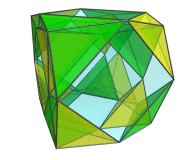
For clarity, we've omitted the cuboctahedral cells, showing only the 16 tetrahedral cells, which are alternately colored so that the animation is easier to follow.
Coordinates
The coordinates of the rectified tesseract are all permutations of coordinates and changes of sign of:
- (0, √2, √2, √2)
These coordinates yield a rectified tesseract of edge length 2, centered on the origin.




