The Square Pyramid
The square pyramid is one of the Johnson solids. It is bounded by 4 equilateral triangles and 1 square, for a total of 5 faces, 8 edges, and 5 vertices. It is the first polyhedron in Norman Johnson's list, and thus bears the label J1.

The square pyramid can be attached to various prisms to form various augmented prisms, such as:
- Augmented triangular prism (J49);
- Biaugmented triangular prism (J50);
- Triaugmented triangular prism (J51);
- Augmented hexagonal prism (J54);
- Parabiaugmented hexagonal prism (J55);
- Triaugmented hexagonal prism (J57).
It can also be attached to the sphenocorona (J86) to make the augmented sphenocorona (J87).
Projections
In order to be able to identify the square pyramid in various projections of 4D objects, it is useful to know how it appears from various viewpoints. The following are some of the viewpoints that are commonly encountered:
| Projection | Envelope | Description |
|---|---|---|
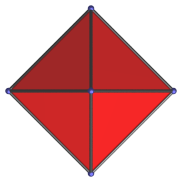 |
Square | Top view (apex-centered parallel projection). |
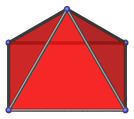 |
Irregular pentagon | Parallel projection centered on triangular face. |
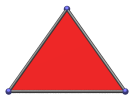 |
Isosceles triangle | Projection parallel to base. The base square face projects to the bottom edge of the projection image. |
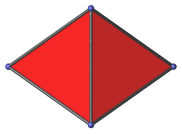 |
Rhombus | Projection centered on a base-to-apex edge. The image of the apex coincides with image of one of the vertices of the base. |
Coordinates
The simplest Cartesian coordinates of the square pyramid, being half of a regular octahedron, are:
- (±1, 0, 0)
- ( 0, ±1, 0)
- ( 0, 0, 1)
These coordinates can be refined to be origin-centered and have edge length 2, thus:
- (±√2, 0, −√2/5)
- (0, ±√2, −√2/5)
- (0, 0, 4√2/5)
Occurrences
The square pyramid is a versatile shape that occurs in many interesting CRF polychora (4D generalizations of the Johnson solids), including (but not limited to):
- Cube antiprism (K4.15);
- Cube atop icosahedron (K4.21);
- The cubical pyramid (K4.26);
- The square magnabicupolic ring (K4.105);
- Octahedron atop rhombicuboctahedron (K4.107);
- The pentagonal prism pyramid (K4.141);
- The octahedral ursachoron;
- The deca-augmented 5,10-duoprism;
- The deca-augmented 5,20-duoprism;
- The biparabigyrated cantellated tesseract;
- The swirlprismatodiminished rectified 600-cell;
- The bilunabirotunda pseudopyramid;
- The triangular hebesphenorotundaeic rhombochoron.




